Linus Tang
Davidson Academy Online
Reno, NV
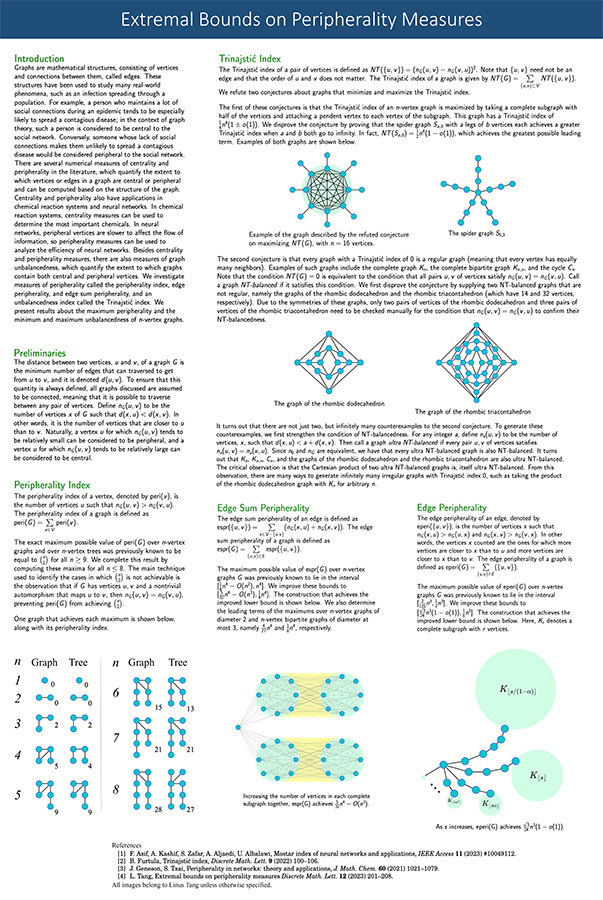
Extremal Bounds on Peripherality Measures
Linus Tang researched centrality and peripherality measures in graph theory for his math project. He improved the best-known bounds on the values attained by several peripherality measures. Applications could include tracing disease-spread and understanding systems of chemical interactions.
View PosterLinus Tang, 17, of San Jose, California, researched peripherality measures in graph theory for his Regeneron Science Talent Search mathematics project. Mathematical networks, made of edges and vertices, are used to model person-to-person interactions to trace disease spread, or to understand systems of chemical interactions, among other applications. A vertex is called central if it is important to the network, such as an influencer in a social network, or a key chemical in a system of reactions.
Peripherality is the opposite of centrality. In his work, Linus researched how central and peripheral the vertices in a network can be. He gave bounds on the values that can be achieved by several numerical measures of peripherality.
Previous researchers had proven such upper and lower bounds and formed conjectures about the graphs that achieve them. Linus proved tighter upper and lower bounds and refuted two of these conjectures.

Linus attends Davidson Academy Online in Reno, Nevada. In 2021, he won first place at the Stanford Math Tournament in the combinatorics round. The son of Seow-Huang Goh and Hsiu-Khuern Tang, Linus spent spring 2022 researching the mathematics behind the game Bulgarian Solitaire.

Beyond the Project
Linus’s favorite strategy game is the board game Go. He loves that Go strategy comes from just a few simple rules but is extremely complex and difficult to master.
FUN FACTS: Linus won his first math competition, called Math Kangaroo, in first grade. He credits the countless hours he spent putting together jigsaw puzzles as a toddler for honing his visualization skills.